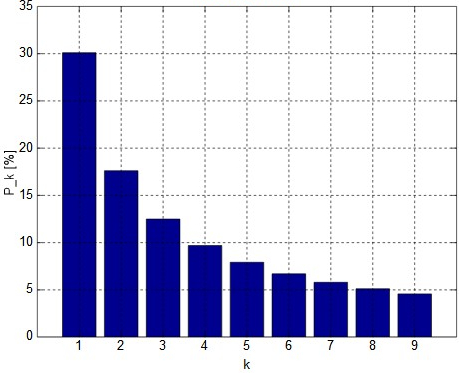
A subject of fascination to mathematicians, Benford’s law states that for many sets of numbers, the first or “leading” digit of each number is not random. Instead, there is a 30.1 per cent chance that a number’s leading digit is a 1. Progressively higher leading digits get increasingly unlikely, and a number has just a 4.6 per cent chance of beginning with a 9.
– NewScientist article
The NewScientist article, linked above, introduced me to Benford’s Law, which is just ridiculous at first glance. Apparently it is a natural consequence of normal distribution data spanning at least a few magnitudes (R.M. Fewster, A Simple Explanation of Benford’s Law). One application of the law is keeping it in mind when analyzing data and looking for forgeries. How do you think the phenomenon would differ in other bases?
Comments
Comment by LG on 2010-10-15 10:27:57 +0000
That’s how a lot of accountants find out if corporations are fibbing on their accounts; people tend to believe that evenly spaced numbers indicate random numbers, but they don’t realize random numbers are really predictable.
Another application is linking the anonymous Federalists papers to Alexander Hamilton via word length computer analysis.
categories:
- read
tags:
- diagram
- math